A few weeks back, I stumbled on this memoir by Alfred Kempe on the theory of mathematical form published in 1886. Here’s a tweetstorm as I read the paper. 

Alfred Kempe was the student of Arthur Cayley. In this philosophical work, he tries to unify geometrical form with logic. It would later influence Royce, Peirce, and a slew of mathematicians in their work on logic. It is available here: royalsocietypublishing.org/doi/10.1098/rs… 

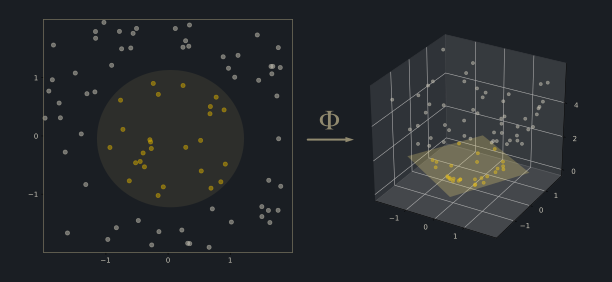
The scope of this memoir is to distinguish the necessary matter of exact thought from its accidental garbs. Kempe believes that algebraical, geometrical, logical, and other kinds of thinking share a unified connected form. 

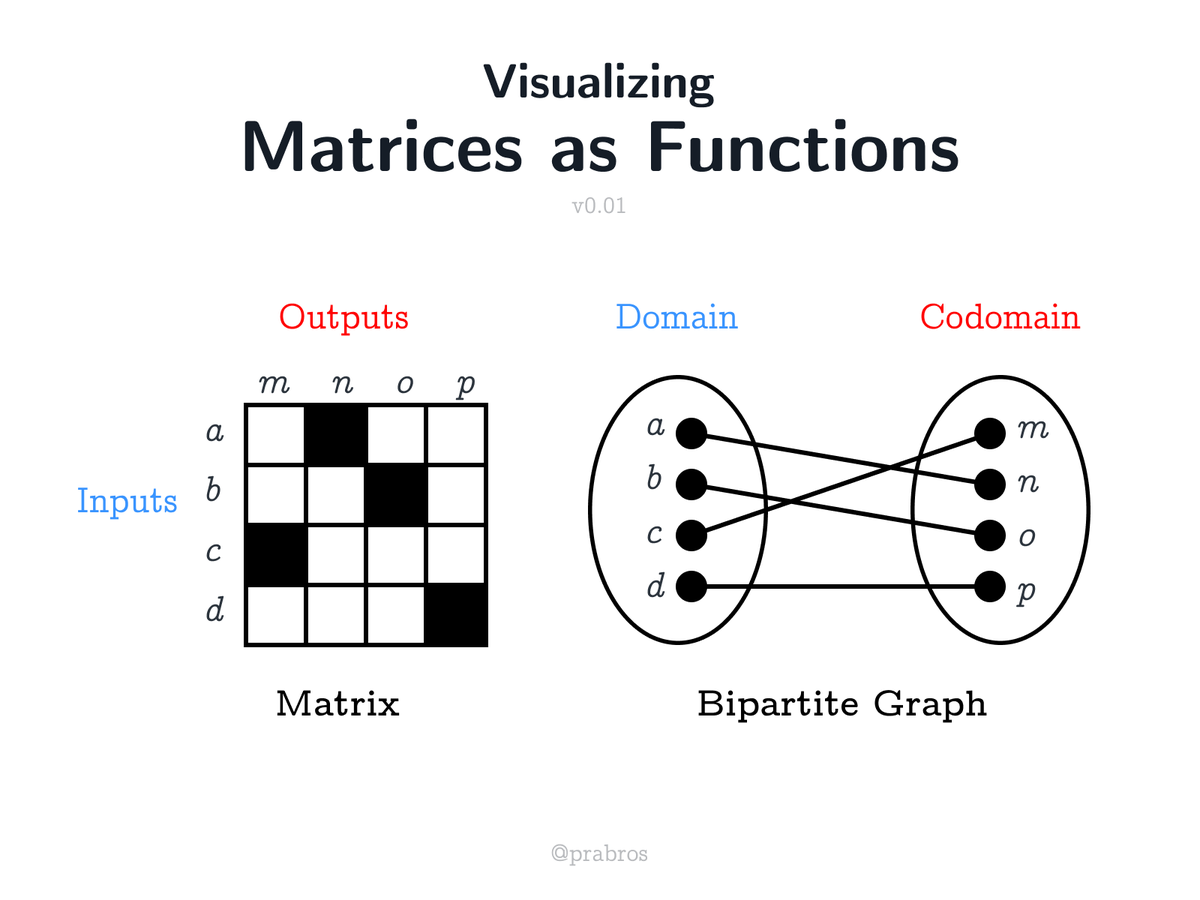
When reasoning about concepts, they are treated as separate entities called units here. The sameness and difference between them is considered as a fundamental principle. 

This principle applies to higher ranks of assembly: When choosing pairwise, you can distinguish between some selections whereas others are the same. 

The same idea applies to choosing units, which can be thought of as the trace in modern mathematical parlance: Selecting b after a (ab) is different from selecting a after b (ba). 

The terminology of collections and aspects are defined: Collections are n-units considered as a single entity, while selections and traces are aspects of this collection. 

Collections assume different forms by the virtue of their component count and the way in which these components are distinguished. Two collections with same component count may have different forms by the way in which their components are distinguished. 

Collections which are undistinguished share the same form while sharing the same form doesn’t mean that they are undistinguished. 

The same form couched in different dressing (notations, frameworks etc.) could lead them to be categorized under different disciplines of science. Kempe noted this pattern early, this has sort of become the norm in modern scientific discourse. 

• • •
Missing some Tweet in this thread? You can try to
force a refresh