Thread by @3blue1brown

I'd like to tell you about a game/puzzle to help celebrate today.

We'll call it "Death's Dice".

(1/9)

Death finds you. You plead with him that it's too soon, and he agrees to a concession. Every year, he'll roll a set of dice, and if it turns up snake eyes (both 1's) he'll take your life, otherwise, you get one more year.

But it's not necessarily a normal pair of dice.

(2/9)

On the first year, both "dice" will only have two sides, numbered 1 and 2. So in that first year, there's a 25% chance of rolling snake eyes and ending things there.

(3/9)

On the second year, he comes with tetrahedral dice, i.e. both are four-sided, numbered 1 through 4, and again only takes your life if he rolls two 1's.

(4/9)

The next year, the dice are six-sided, after that, eight-sided, etc., etc.

Each year you have a lower and lower chance of dying, but he'll come back every year with a new set of dice, never stopping.

(5/9)

You might think the question now is something like "what's your expected number of remaining years of life?"

But actually, Death gave you a pretty good deal.

(6/9)

The better question to ask here is "what's the probability that you end up immortal?" That is, the probability that Death rolls infinitely many times, with his ever-growing dice, and never once turns up snake eyes.

(7/9)

Stop reading now if you don't want the answer spoiled.

Empirically, if you go run some simulations (which I encourage you to do!), you may notice that no matter how many years you run this, you're probability of survival never seem to drop below around 0.6366...

(8/9)

But what is this value?

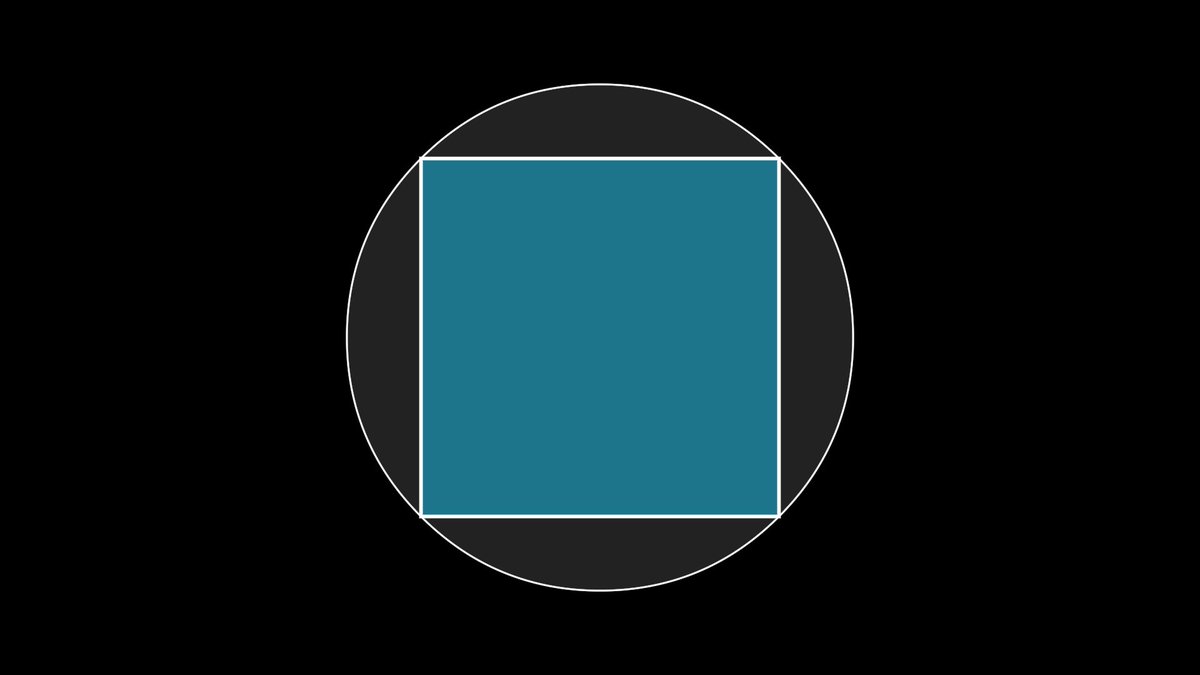
As it happens, your chances of immortality are precisely 2/π

This probability is also the square-to-circle area ratio below. Almost certainly coincidence, but extra points to anyone with a logical link between the puzzle and this diagram.

Happy pi day!