Share this page!

Enter URL or ID to Unroll

You can paste full URL like: https://x.com/threadreaderapp/status/1644127596119195649
or just the ID like: 1644127596119195649

How to get URL link on X (Twitter) App

  1. On the Twitter thread, click on or icon on the bottom
  2. Click again on or Share Via icon
  3. Click on Copy Link to Tweet
  4. Paste it above and click "Unroll Thread"!
  5. More info at Twitter Help
David Salazar Profile picture
David Salazar
@DavidSalazarVir
Dilettant'n Data Science. I enjoy multiplying random numbers over and over again. #rstats and Python.
3 subscribers
Sep 7, 2020 • 6 tweets • 3 min read
Smell BS in election models?

Well, most of them are way too confident and too volatile.

@nntaleb shows that one cannot invoke probabilistic thinking in one's forecasts of a highly uncertain election unless the forecasts are stable and around 50/50. Why?

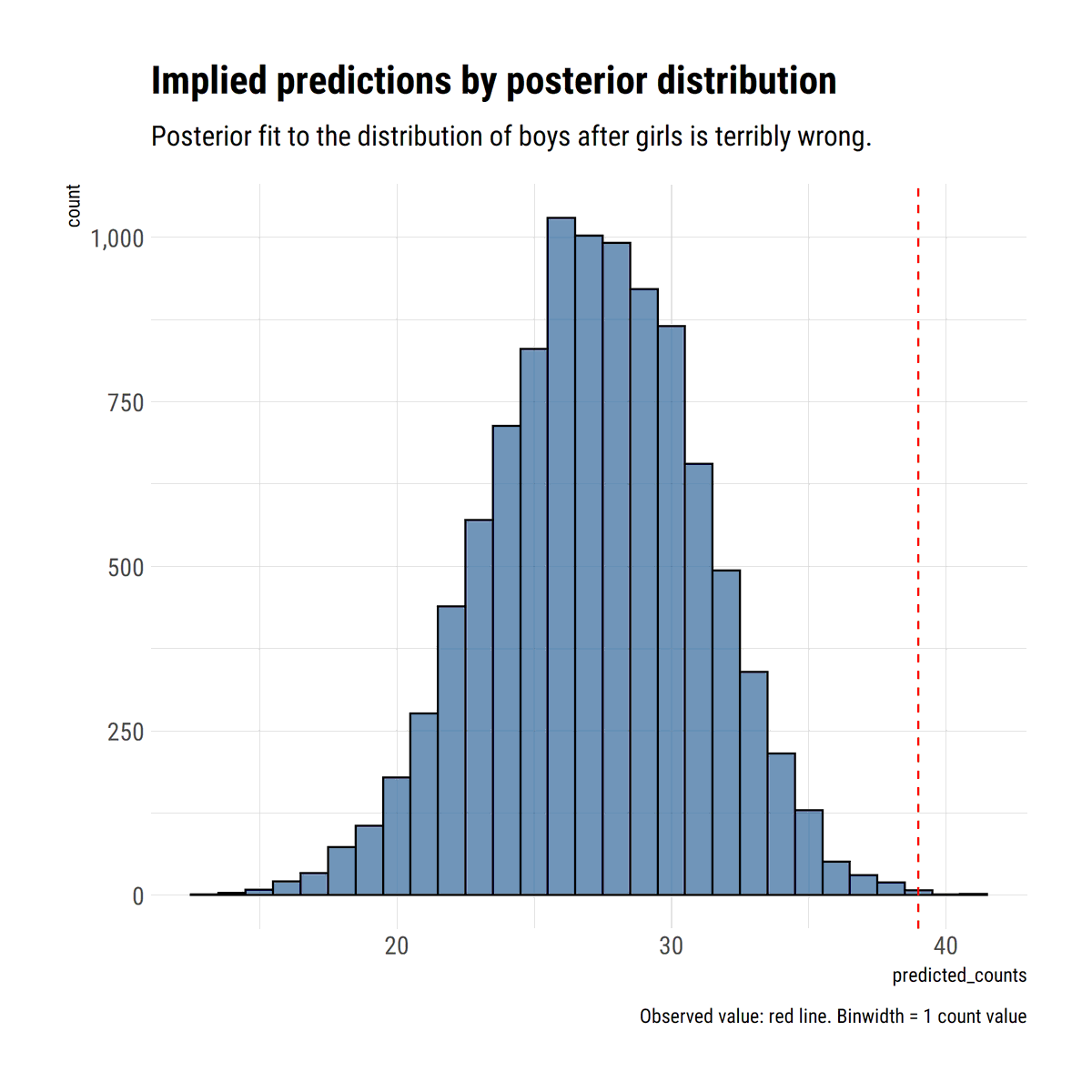
Explanation⬇️ Image With a single event, frequency calibration is meaningless. Yet there are other ways to ensure coherence in one's belief.

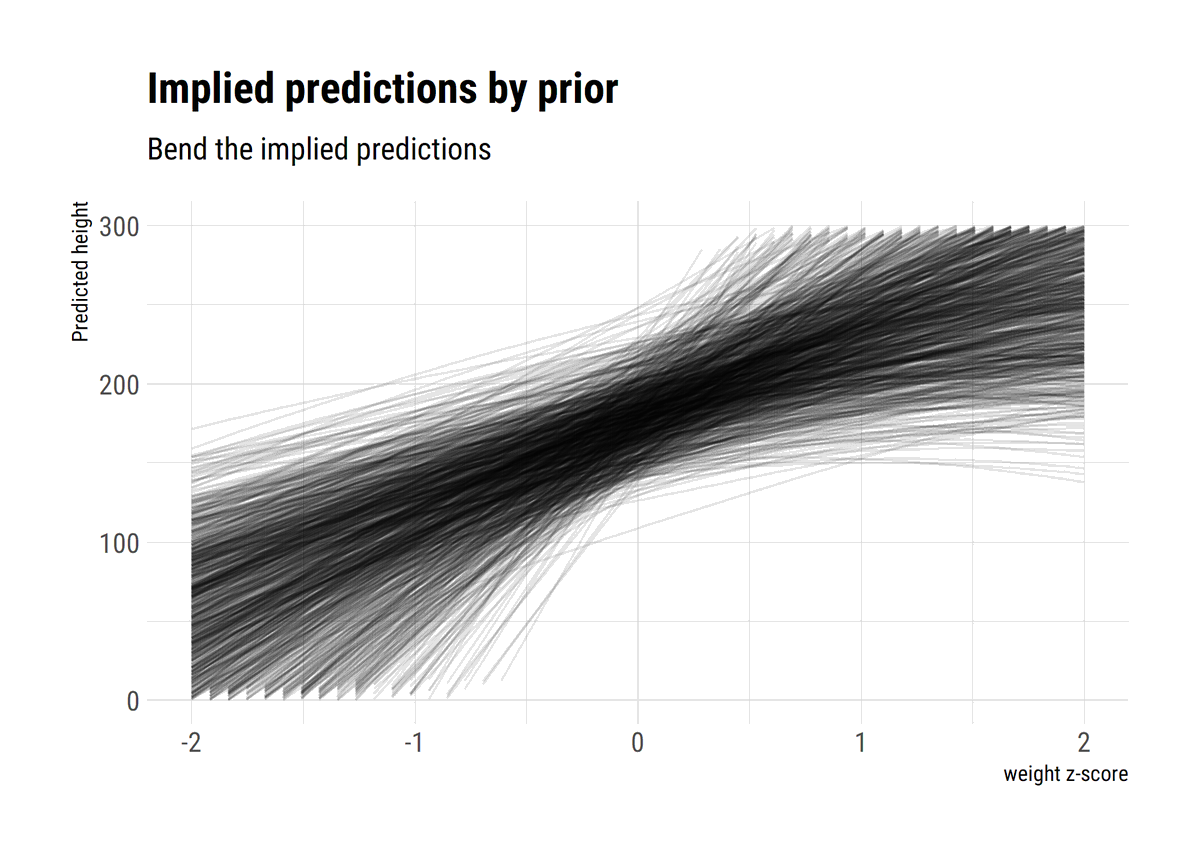
De Finetti showed that coherent subjective probabilistic statements must imply a bet that precludes the possibility of arbitrage. Excerpt from De Finetti⬇️ Image
Jul 20, 2020 • 33 tweets • 20 min read
I find stats talk around causality wanting: most of the time, the concept is simply side-stepped. To remedy this, I'll read through @yudapearl's seminal work 'Causality' using his 'Book of Why' as an interpretative key. In this thread, I'll share what I learn. Bayesian Networks and Probability Distributions. How can we model a joint probability distribution in a computer? What is the probabilistic connection? d-separation and testable implications from graphical methods, Ladder of Causation..

Blogpost:
david-salazar.github.io/2020/07/18/cau…
#rstats
Apr 23, 2020 • 6 tweets • 7 min read
Going over @rlmcelreath's latest book in #rstats. However, I am using #tidyverse tools. So far, I have watched the lectures, completed homework for week 1 and the exercises in chapter 2 of the book. Hope to make it till the end!
Btw, the book is amazing.
david-salazar.github.io/2020/04/19/sta… ImageImage Week 2 of Statistical Rethinking! Lectures and homework completed. @rlmcelreath is equal parts entertaining and knowledgeable. Homework made crystal clear how difficult it is to set priors for polynomial models #tidyverse #rstats

david-salazar.github.io/2020/04/28/sta… ImageImage
Apr 23, 2020 • 55 tweets • 33 min read
Trying to get my head around fat-tails by studying @nntaleb's latest technical book. Replicated some plots in #rstats #tidyverse
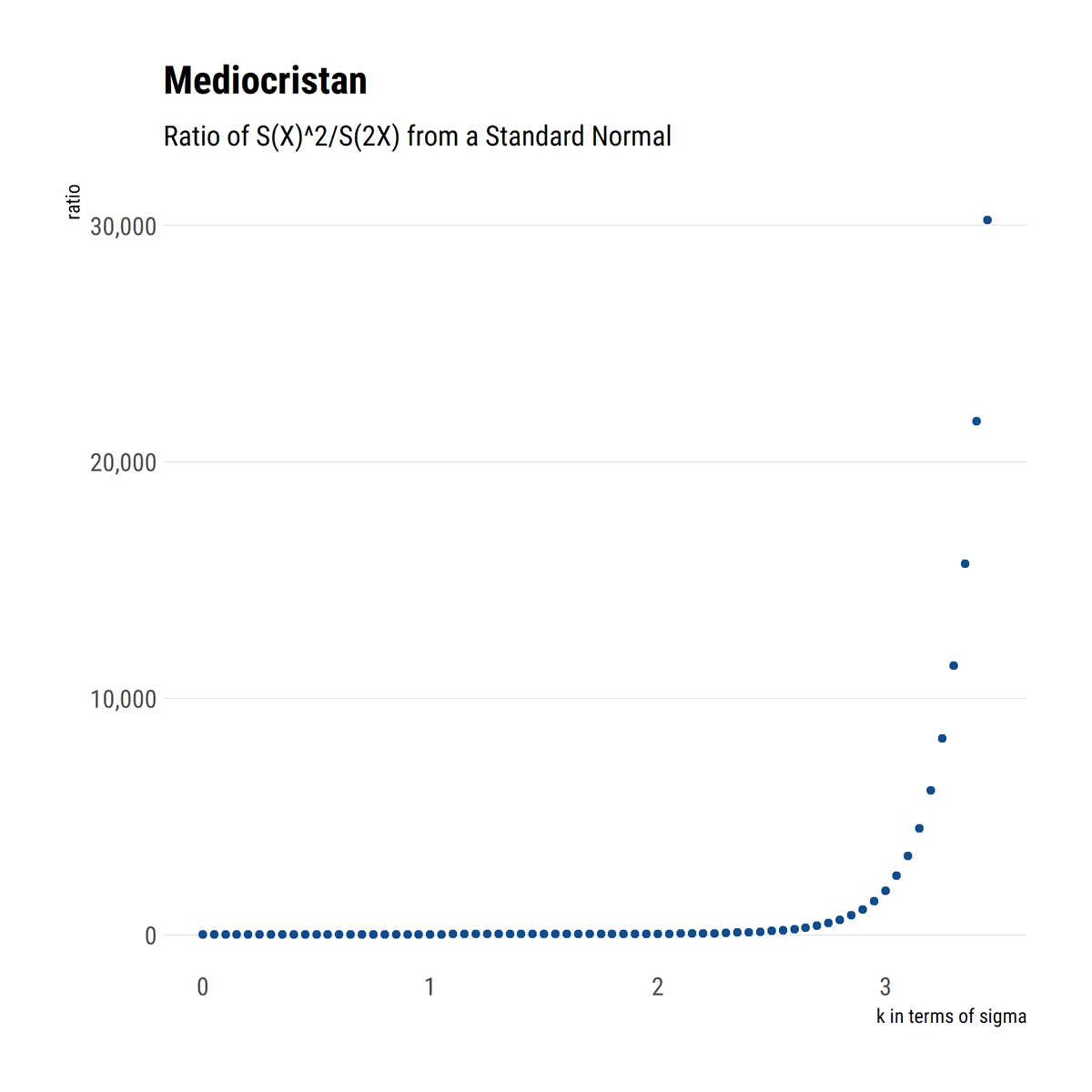
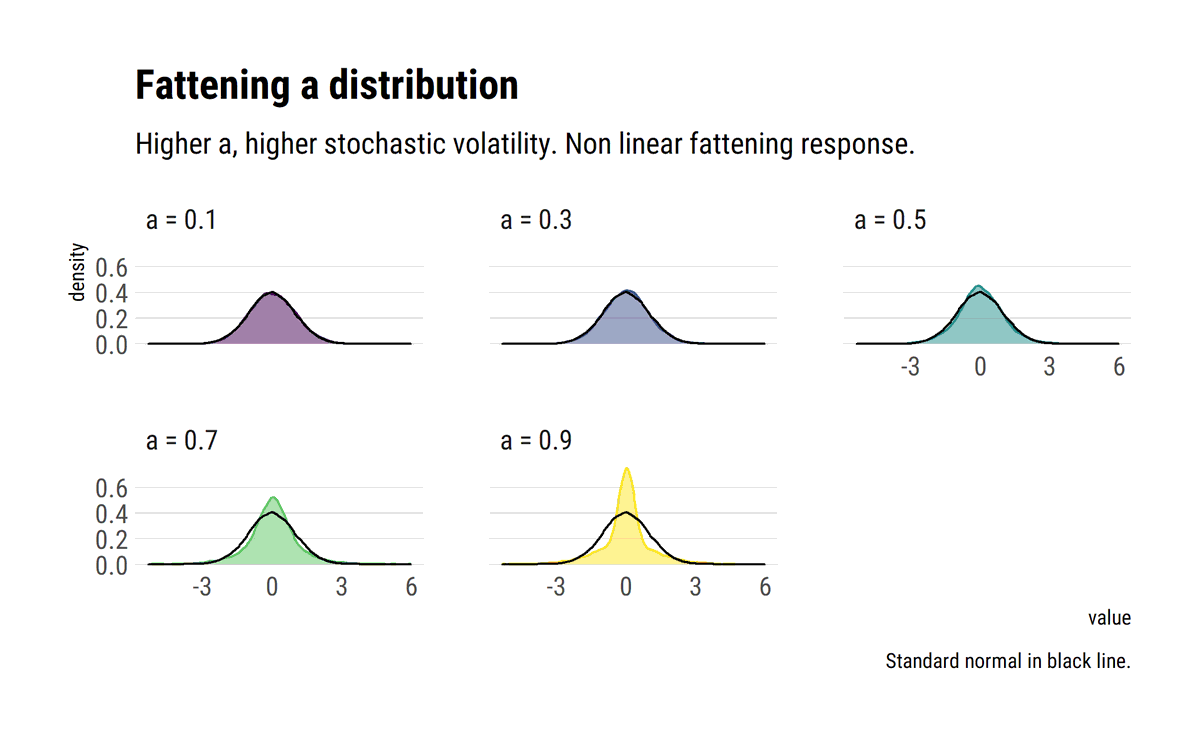
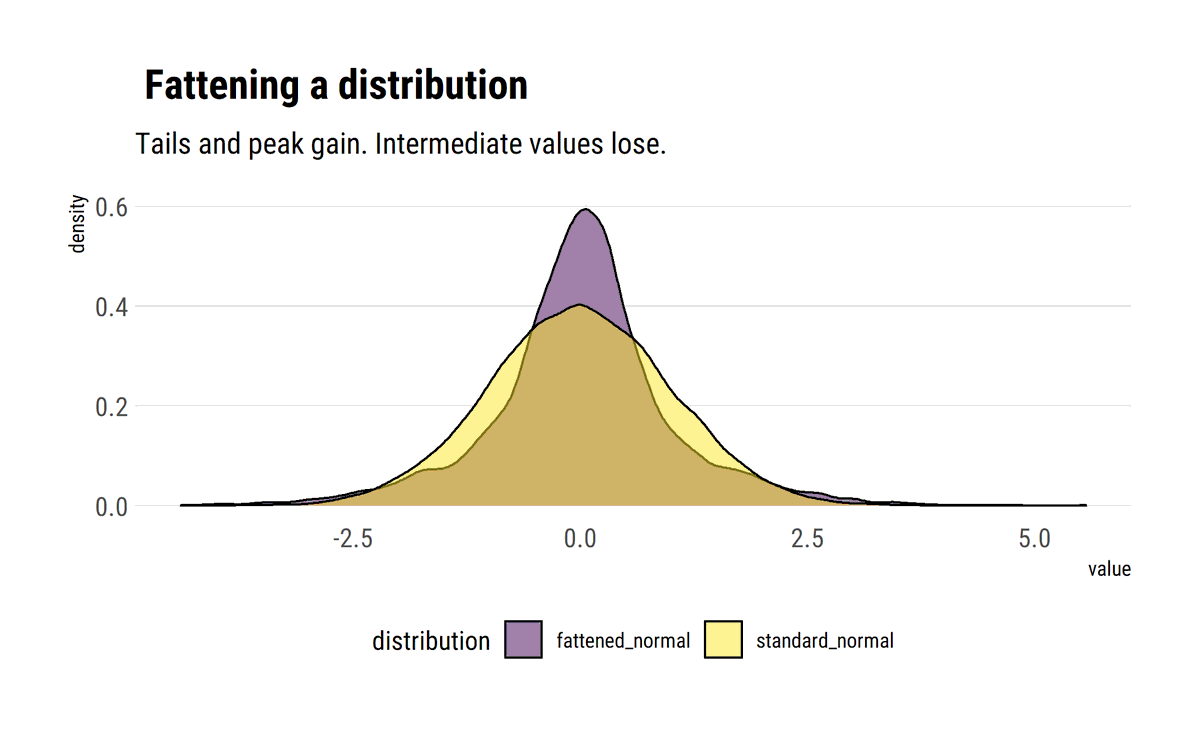
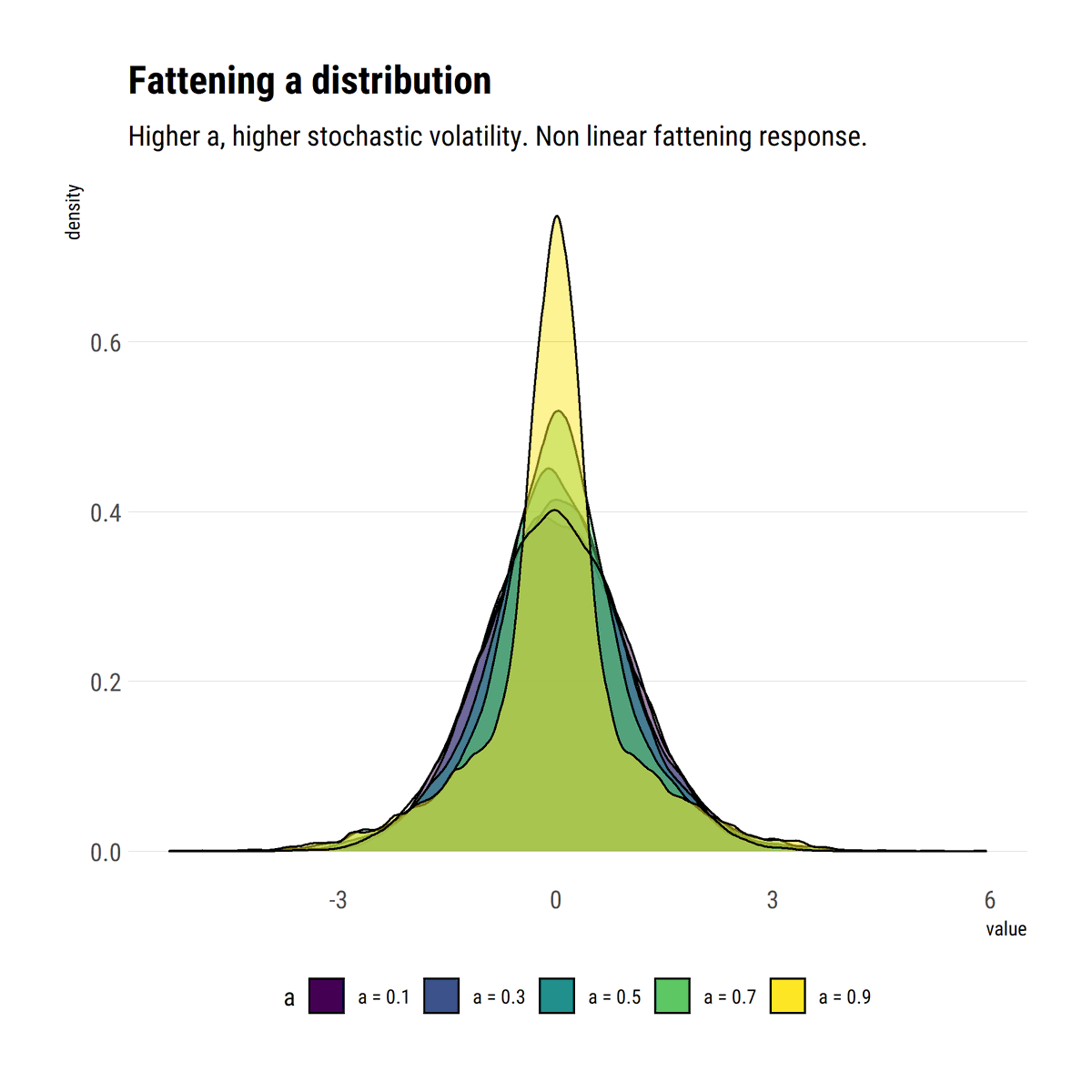
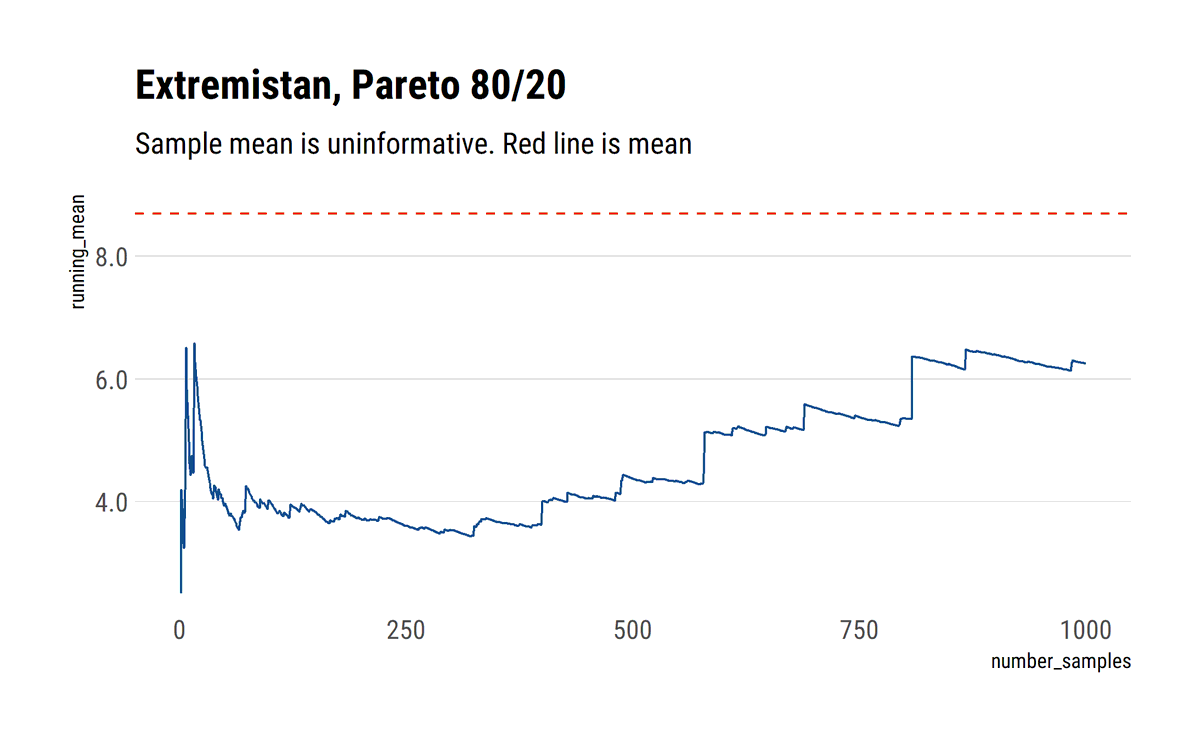david-salazar.github.io/2020/04/17/fat… #rstats and @nntaleb's work. By fattening the tails, one learns that the tail events are convex to the scale of the distribution. Thus, the problem compunds: tail events have an increasingly large role, but we cannot estimate their probabilities reliably

david-salazar.github.io/2020/05/09/wha…