I should leetcode and go back to sw dev, markets are whimsical send tweet A decade in this industry and the best investment thesis I can come up with is “rates won’t explode higher and equities will probably do okay”

Its been a couple years since I did an explainer of how an autocallable structured product works, so lets walk through this relatively straightforward one from Morgan Stanley. Autocallables are extremely popular across the globe because of the high coupons morganstanley.com/structuredinve… Let’s start with the coupons*. They’re not really coupons. They’re ‘contingent coupons’ which are complex triple binary options. All assets have to be above some level for you to be paid the monthly coupons equivalent to a ~9% coupon. The dealer takes corr and binary option risk

FYI if you want to find the filings for the Infinity Q Diversified Alpha fund, you have to search on EDGAR for CIK 0001261788 From there you have to click the “List all funds” link and go to the Diversified Alpha fund

Some mornings I wake up and think about Carr Wu 2004, a great options paper I may be misremembering about static hedging of option portfolios. engineering.nyu.edu/sites/default/… The usual Black Scholes world says that an arbitrary European option payoff can be replicated by continuous time dynamic delta hedging

Some people here like @jsoloff are big fans of stochastic calculus so I here are some fun facts on Wiener processes, Ito's lemma, Feynman-Kac, backward Kolmogorov, and the Black-Scholes PDE Going back to basics, the Wiener process is a continuous-everywhere, differentiable-nowhere stochastic process, that is the limit of an infinitely fast random walk. You flip coins infinitely fast and it becomes continuous. (Note the notation and math I do here is handwavey)

I'm bored and people seem to like @bennpeifert vol explainers so here's a dollar store version of Shreve and Bjork's intro to stochastic calc for option pricing. A very rough guide to learning one way Black Scholes is derived The simplest stochastic process is a random walk. You flip coins and either go +1 or -1.

Now how do the big boys do this in the real world? They use @AxiomaInc or @MSCI_Inc (formerly called MSCI BARRA but I think they dropped the name)

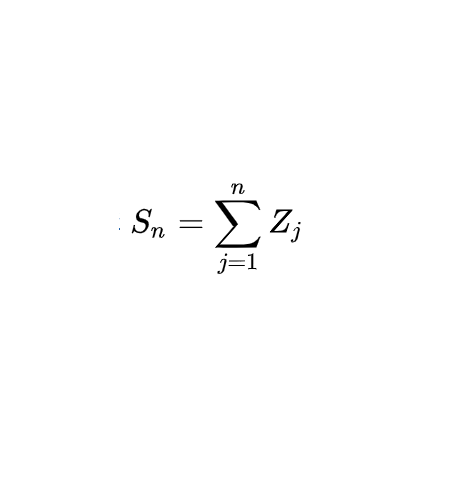
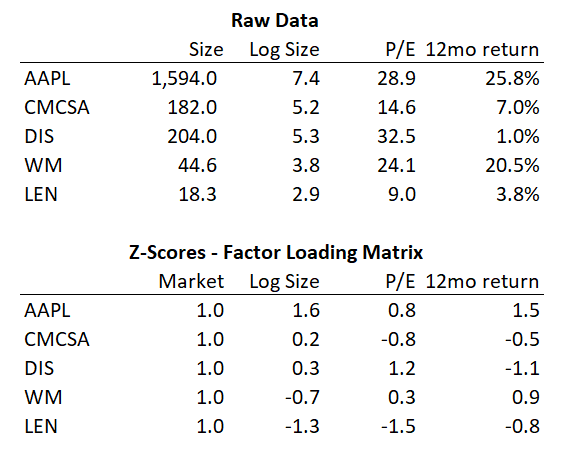
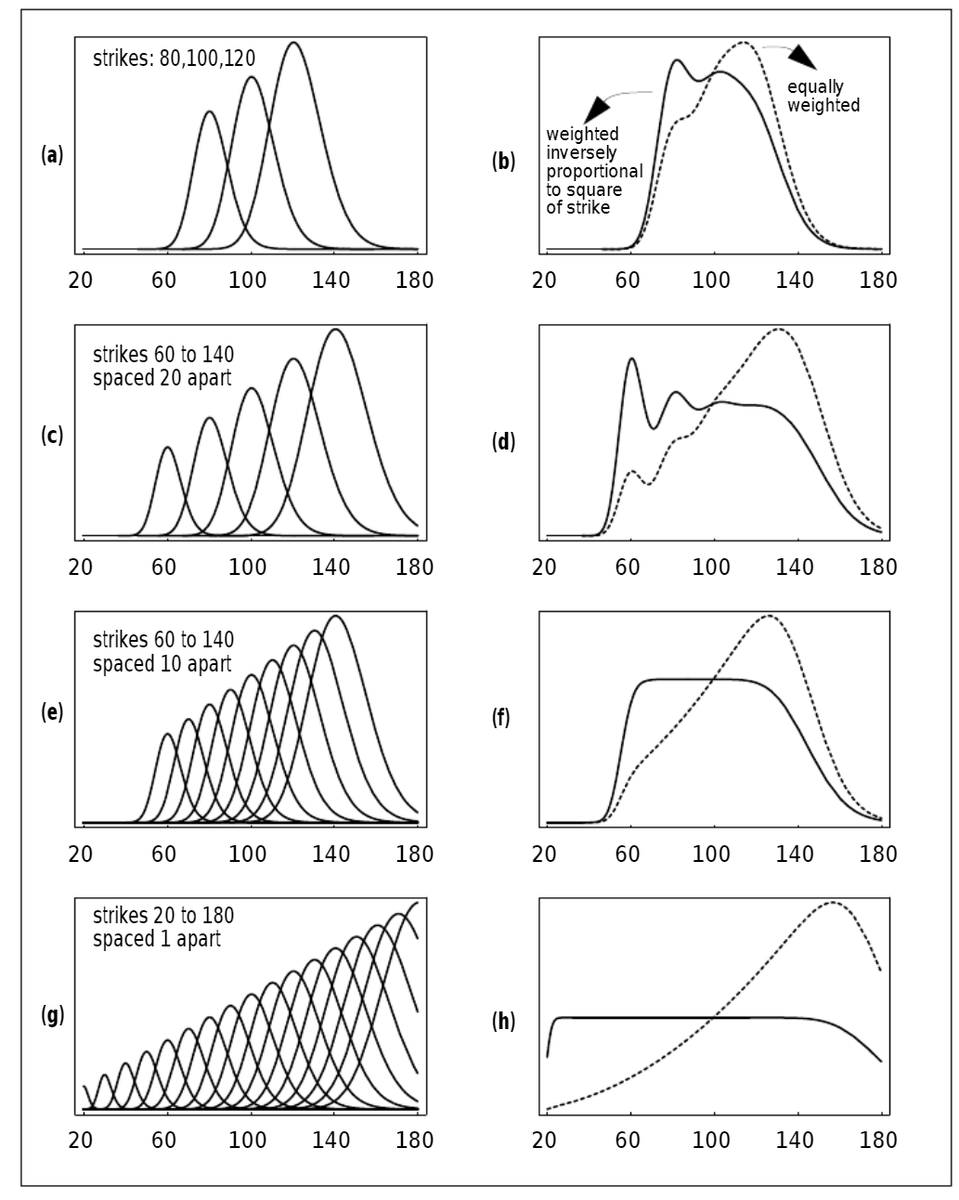
https://twitter.com/ONAN_OUS/status/1278722283519873024

The main thing is we want a closed-form way to calculate factor exposures and the returns of those factors. We first start by defining our factors and their metrics.

So now we have size, value, momentum, quality, low volatility factors. What do we do with them?

https://twitter.com/ONAN_OUS/status/1278719269996425216

The push to bring these into practice was done at Goldman Sachs Asset Management. @CliffordAsness started there in 1994, and left to create AQR in 1998. Mark Carhart ran the Global Alpha fund at GSAM until about 2010 and left to launch Kepos with Bob Litterman, another GSAM alum.

ONAN story time again – quant equity. This is completely irrelevant to the gigantic macro issues of the day, so feel free to waste your time in a journey into multiplying matrices. Arguably one of the first people to do this was Ed Thorpe – in his book at the very end he mentions starting a systematic investing effort though the fund got wound down before it really took off. amazon.com/dp/B07ZWJFYW5

As your resident vol explainer guy, here's a long-form version of how one can be down 200% a la Malachite. They were doing capped vs. uncapped variance swaps, 'picking up $100 bills in front of a Soviet locomotive' Variance swaps are technically exotic derivatives and are nonlinear, but they can be hedged easily using vanilla options, so they aren't all that exotic. @EmanuelDerman wrote the holy grail on them researchgate.net/publication/33…