
Digital Geometer, Assoc. Prof. of Computer Science & Robotics @CarnegieMellon @SCSatCMU and member of the @GeomCollective. There are four lights.
3 subscribers
How to get URL link on X (Twitter) App


 A similar thing happens when (many) people learn linear algebra:
A similar thing happens when (many) people learn linear algebra:

 *Of course I do in reality know why people use this diagram: it fits into a common visual language used for neural networks.
*Of course I do in reality know why people use this diagram: it fits into a common visual language used for neural networks.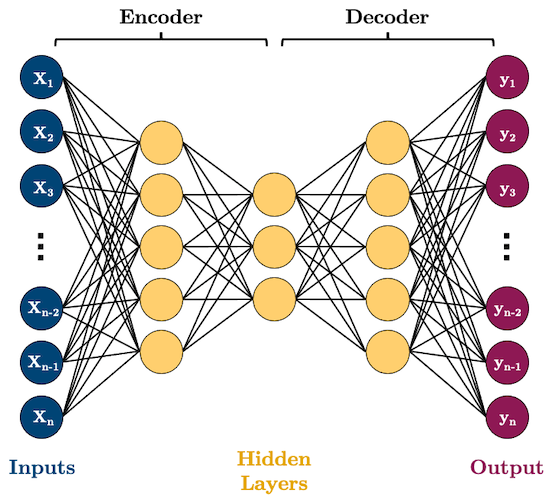

 Here's the paper, which is an industry-funded collaboration between my PhD student Hossein Baktash at @SCSatCMU, @nmwsharp at @nvidia, and Qingnan Zhou & @_AlecJacobson at @AdobeResearch.
Here's the paper, which is an industry-funded collaboration between my PhD student Hossein Baktash at @SCSatCMU, @nmwsharp at @nvidia, and Qingnan Zhou & @_AlecJacobson at @AdobeResearch.




 In particular:
In particular:
 𝗥𝗲𝗽𝘂𝗹𝘀𝗶𝘃𝗲 𝗦𝗵𝗮𝗽𝗲 𝗢𝗽𝘁𝗶𝗺𝗶𝘇𝗮𝘁𝗶𝗼𝗻
𝗥𝗲𝗽𝘂𝗹𝘀𝗶𝘃𝗲 𝗦𝗵𝗮𝗽𝗲 𝗢𝗽𝘁𝗶𝗺𝗶𝘇𝗮𝘁𝗶𝗼𝗻https://twitter.com/keenanisalive/status/1468011750608056324

 Harmonic functions are everywhere in geometric & visual computing, as well as in math, engineering, and physics. So, it's pretty powerful to be able to visualize them directly.
Harmonic functions are everywhere in geometric & visual computing, as well as in math, engineering, and physics. So, it's pretty powerful to be able to visualize them directly.

https://twitter.com/KangarooPhysics/status/1625423951156375553
 [2/n] To be clear, this 🧵 isn't about finding a better packing—or even finding it faster. Wizards like @KangarooPhysics surely have better tricks up their sleeves 🪄
[2/n] To be clear, this 🧵 isn't about finding a better packing—or even finding it faster. Wizards like @KangarooPhysics surely have better tricks up their sleeves 🪄


 Our #SIGGRAPH2022 paper takes a major step toward this vision by building a bridge between PDEs & volume rendering: cs.dartmouth.edu/wjarosz/public…
Our #SIGGRAPH2022 paper takes a major step toward this vision by building a bridge between PDEs & volume rendering: cs.dartmouth.edu/wjarosz/public…








 We've already tried a few dozen methods—from classics like "KnotPlot", to baselines like L-BFGS, to bleeding-edge algorithms like AQP, BCQN, etc.
We've already tried a few dozen methods—from classics like "KnotPlot", to baselines like L-BFGS, to bleeding-edge algorithms like AQP, BCQN, etc.

