Get a cup of coffee.
In this thread, I'll walk you through the basics of portfolio diversification.
Imagine that you're a journalist.
You just scored an interview with the world's greatest investor.
You're really excited.
It's the day of the interview.
You're escorted into the great man's office.
He's seated behind his desk. He greets you warmly. You exchange pleasantries.
It's time to begin the interview.
Noticing your puzzled look, the investor explains:
This is a magic scrabble set. Every time you open the box, a few letters jump out and arrange themselves into a stock ticker. After a few seconds, they jump back into the box.
This is how the investor picks all his stocks.
There's just one catch: stocks picked using this method are not automatic slam dunks.
Instead, each stock suggested by the scrabble set either doubles or halves over the next 1 year. There's a 50/50 chance of either outcome, and no way to predict it in advance.
Because the investor has grown fond of you (and because he wants you to write a favorable article), he gives you this magic scrabble set for 1 day.
You can use it as many times as you like during this 1 day, but after that you have to return it back to the investor.
The question now is: how do you make the most of your day with the magic scrabble set?
You're not this ultra-rich investor. You're just a poor journalist. So you only have $1M to invest in stocks.
You *could* roll the die on just 1 stock.
That is, you open the box just once, note down the magic stock ticker, put your entire $1M into that stock, and hold for 1 year.
What will happen to your $1M if you followed this "1 stock" strategy?
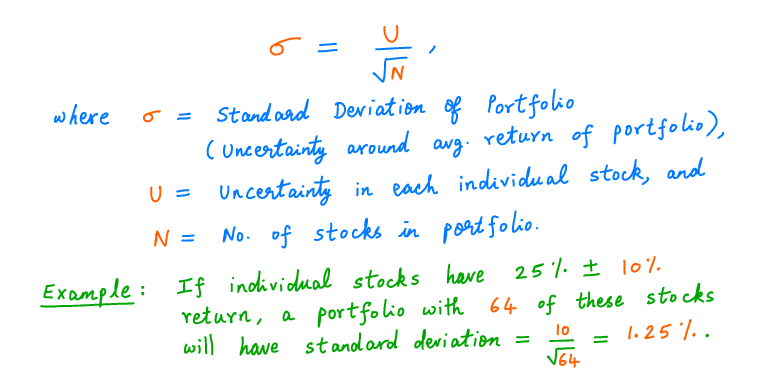
You may have heard of a math concept called "standard deviation".
This is just a fancy way of saying "uncertainty around the average".
Unsurprisingly, for the 1 stock strategy, the standard deviation works out to exactly 75% -- the same as our "U" above.
What happens if you "diversify" by using the magic scrabble set more than once?
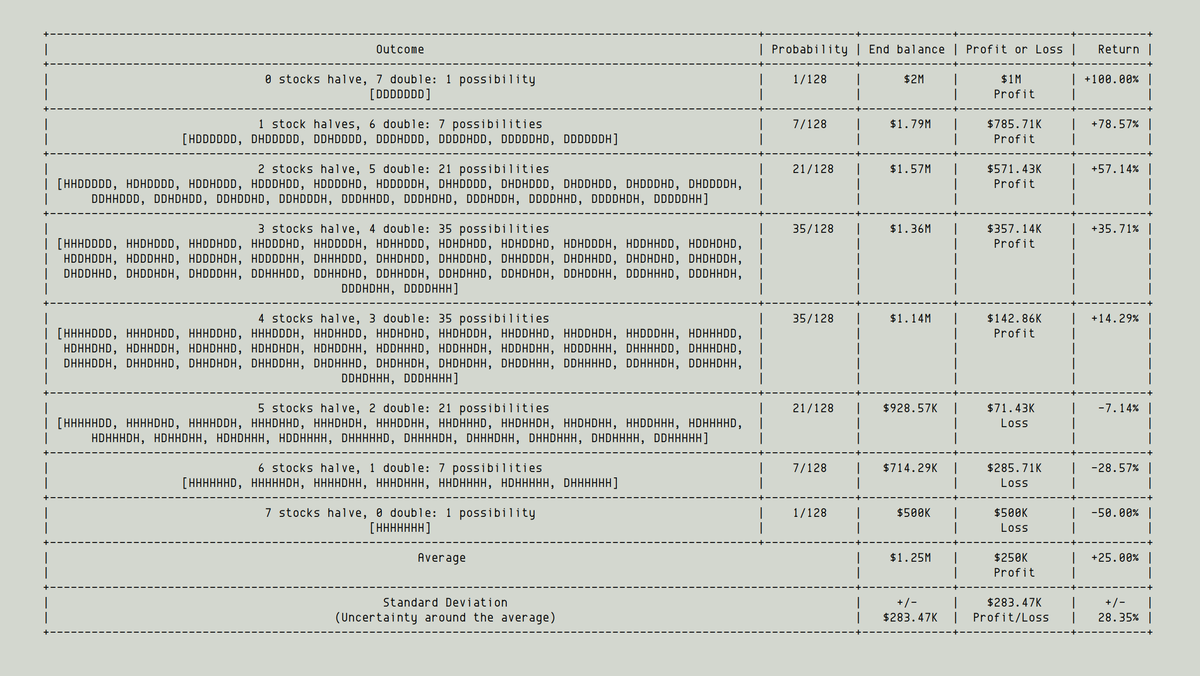
Let's say you open the box 3 times. This gives you 3 stock tickers. You divide your $1M equally among these 3 stocks.
With the "3 stock" strategy, there are many possibilities.
For example, all 3 stocks could Double. This we denote by DDD.
Or Stock 1 could get Halved while Stocks 2 and 3 Double: HDD.
Or Stocks 1 and 3 could Double while Stock 2 is Halved: DHD.
And so on.
As the table shows, whether you invest in just 1 stock or 3 stocks, your *average* return is the same: +25%.
But if you diversify your capital among 3 stocks, your *standard deviation* (the uncertainty around your average return) drops from 75% to 43.3%.
Key lesson 1: Diversifying your capital among many similar bets helps you keep the average return you'd expect from an individual bet, while at the same time reducing the uncertainty around this average return.
In other words, diversification minimizes "tail risk": the chances of getting a return that's far from average (either far better or far worse).
For example, going from 1 to 3 stocks, your chances of a 50% loss go down from 1/2 to 1/8. So do your chances of a 100% gain.
Key lesson 2: There's a law of diminishing returns to the benefits of diversification.
For example, adding just 2 stocks (going from 1 to 3) reduced your standard deviation by ~32% (from 75% to ~43%). But the next 4 stocks only reduced your standard deviation by ~15%.
Another factor to consider:
We started with a pretty extreme kind of bet: Double or Halve. That's 25% (R) +/- 75% (U).
What if our individual bets were less extreme? Say, U = 50% instead of 75%. That is, "Gain Three-Fourth or Lose One-Fourth" instead of "Double or Halve".
Key lesson 3: When your individual bets are more certain, you can afford to make fewer bets.
For example, see the table above. With U = 50% (low uncertainty), it takes just 25 stocks to get to 10% standard deviation. But with U = 75% (high uncertainty), it takes ~50 stocks.
Also, you can see the law of diminishing returns clearly.
For example, for all Us shown, there's less than half a percent benefit when going from a 2500 stock portfolio to a 5000 stock portfolio.
It's clear that if you choose your individual bets carefully (so they have a high degree of certainty), you don't need very many bets.
For example, to achieve a 5% portfolio wide standard deviation, you need just 4 stocks -- provided there's only 10% uncertainty in each.
This is why investors like Buffett can run extremely concentrated portfolios and still be able to sleep well at night.
They're that confident in the certainty of their individual bets. They understand their portfolio companies that well.
Of course, you may not have such high confidence in your analysis/understanding of companies.
This is common, especially if you're new to investing.
This is perfectly OK. Just make sure you have the right level of diversification in your portfolio.
One more thing: the formulas/tables above only apply if your individual bets are independent (uncorrelated).
If your stocks are correlated (ie, the underlying businesses are tied to the same things, like the US economy), you need to diversify more than these tables predict.
Congrats! You've reached the end of another one of my long threads.
Thanks for reading! Enjoy your weekend! Stay diversified!
/End