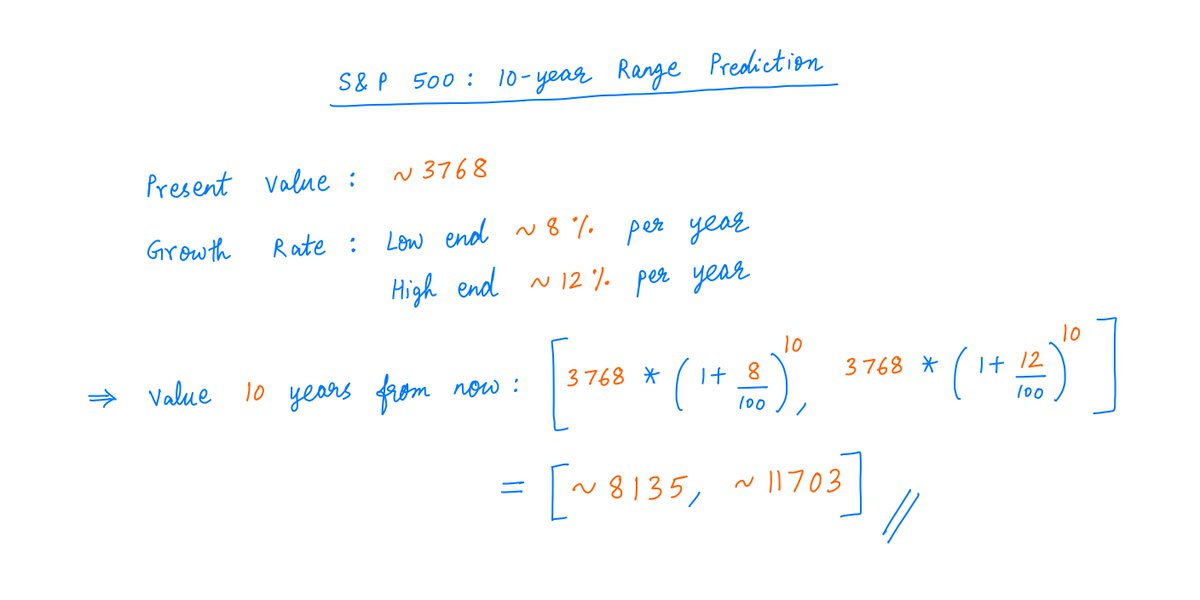1/
Get a cup of coffee.
In this thread, I'll help you understand the basics of Binomial Thinking.
The future is always uncertain. There are many different ways it can unfold -- some more likely than others. Binomial thinking helps us embrace this view.
Get a cup of coffee.
In this thread, I'll help you understand the basics of Binomial Thinking.
The future is always uncertain. There are many different ways it can unfold -- some more likely than others. Binomial thinking helps us embrace this view.
2/
The S&P 500 index is at ~3768 today.
Suppose we want to predict where it will be 10 years from now.
Historically, we know that this index has returned ~10% per year.
If we simply extrapolate this, we get an estimate of ~9773 for the index 10 years from now:
The S&P 500 index is at ~3768 today.
Suppose we want to predict where it will be 10 years from now.
Historically, we know that this index has returned ~10% per year.
If we simply extrapolate this, we get an estimate of ~9773 for the index 10 years from now:
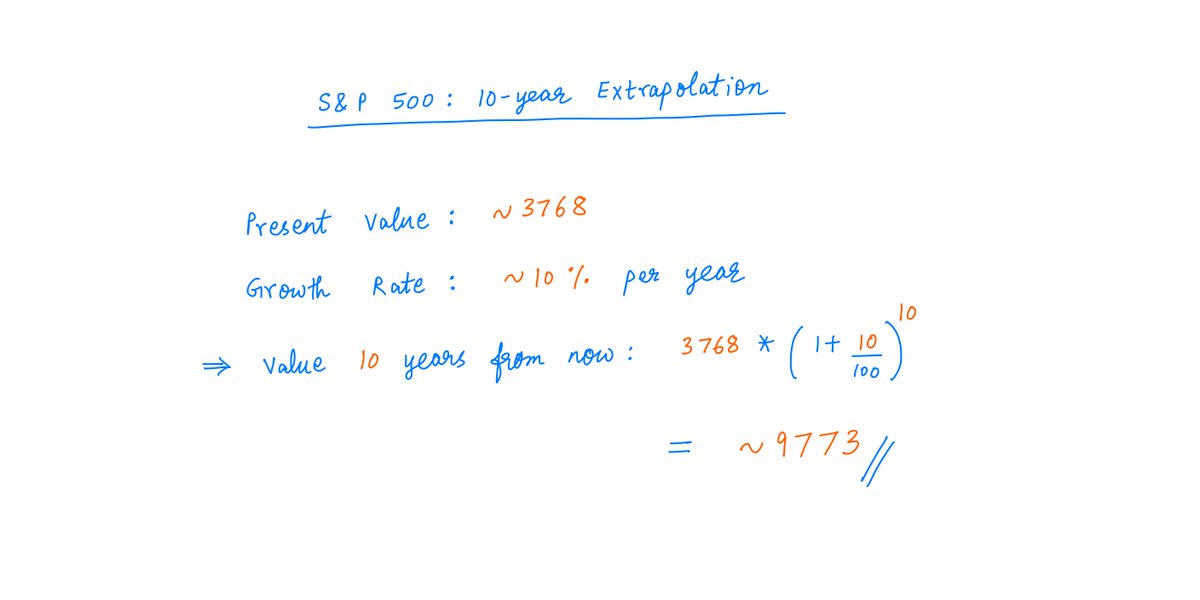
3/
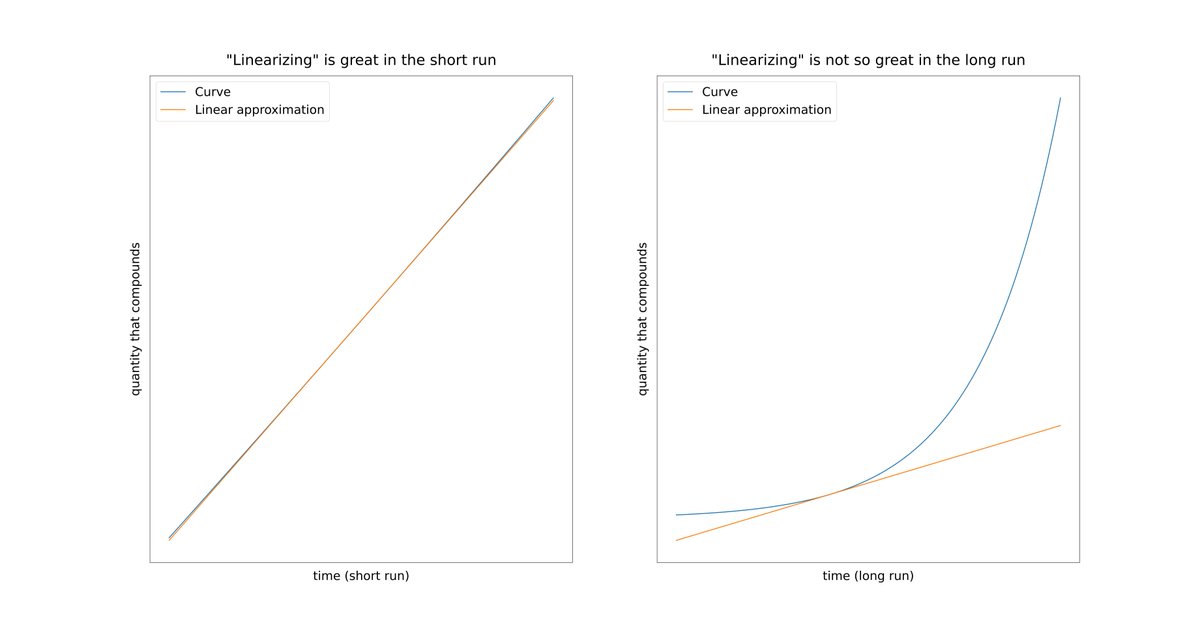
What we just did is called a "point estimate" -- a prediction about the future that's a single number (9773).
But of course, we know the future is uncertain. It's impossible to predict it so precisely.
So, there's a sense of *false precision* in point estimates like this.
What we just did is called a "point estimate" -- a prediction about the future that's a single number (9773).
But of course, we know the future is uncertain. It's impossible to predict it so precisely.
So, there's a sense of *false precision* in point estimates like this.
4/
To emphasize the uncertainty inherent in such predictions, a better approach is to predict a *range* of values rather than a single number.
For example, we may say the index will return somewhere in the *range* of 8% to 12% (instead of a fixed 10%) per year.
To emphasize the uncertainty inherent in such predictions, a better approach is to predict a *range* of values rather than a single number.
For example, we may say the index will return somewhere in the *range* of 8% to 12% (instead of a fixed 10%) per year.
6/
A range is more nuanced than a point estimate, but we still have a problem:
Range estimates tell us nothing about the relative likelihoods of different parts of the range.
For example, which is more likely -- the low end or high end of the range? We don't know.
A range is more nuanced than a point estimate, but we still have a problem:
Range estimates tell us nothing about the relative likelihoods of different parts of the range.
For example, which is more likely -- the low end or high end of the range? We don't know.
7/
Ideally, we want to say something like:
10 years from now, there's a ~93% chance that the index will lie in the range [5648, 15244], and there are 60/40 odds favoring the bottom half of this range.
Binomial thinking enables us to make such *probabilistic* predictions.
Ideally, we want to say something like:
10 years from now, there's a ~93% chance that the index will lie in the range [5648, 15244], and there are 60/40 odds favoring the bottom half of this range.
Binomial thinking enables us to make such *probabilistic* predictions.
8/
With binomial thinking, we can derive not just a *range* of possible outcomes, but also the *probability* of seeing each outcome in this range.
It's easiest to illustrate this with an example.
With binomial thinking, we can derive not just a *range* of possible outcomes, but also the *probability* of seeing each outcome in this range.
It's easiest to illustrate this with an example.
9/
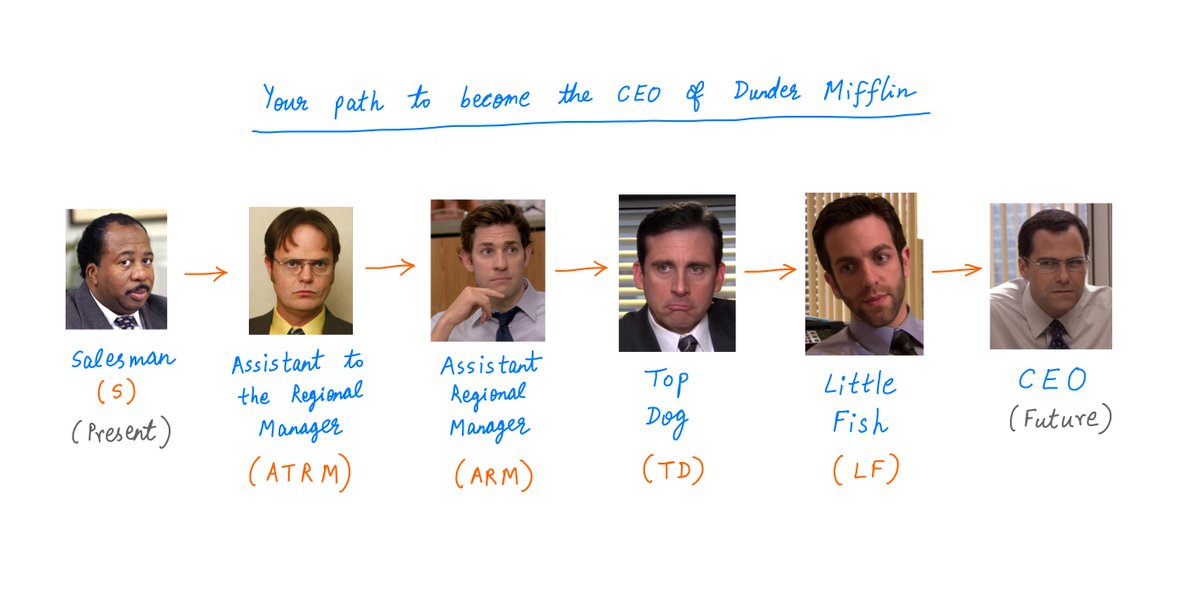
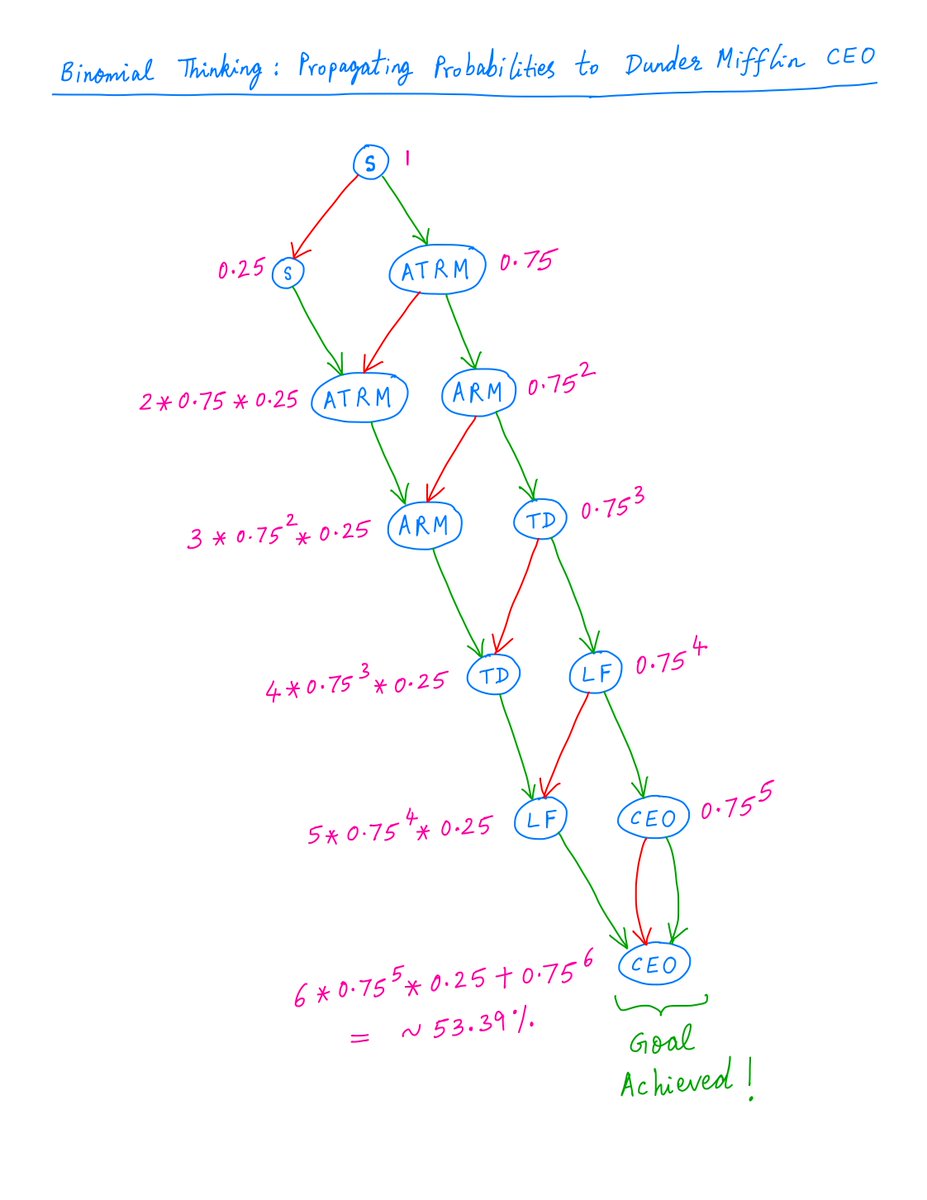
Imagine that you just joined the Dunder Mifflin Paper Company as a Salesman.
From these humble beginnings, you hope to rise quickly within the organization.
You want to become the CEO in 6 years time.
Here's your path to the top job:
Imagine that you just joined the Dunder Mifflin Paper Company as a Salesman.
From these humble beginnings, you hope to rise quickly within the organization.
You want to become the CEO in 6 years time.
Here's your path to the top job:
10/
So, you need 5 promotions to become the CEO.
Let's say you come up for a promotion every year.
And every year, there's a 75% chance you'll get the promotion (and a 25% chance you won't).
So, what's the probability that you'll achieve your goal of becoming CEO in 6 years?
So, you need 5 promotions to become the CEO.
Let's say you come up for a promotion every year.
And every year, there's a 75% chance you'll get the promotion (and a 25% chance you won't).
So, what's the probability that you'll achieve your goal of becoming CEO in 6 years?
11/
Let's tackle this one year at a time.
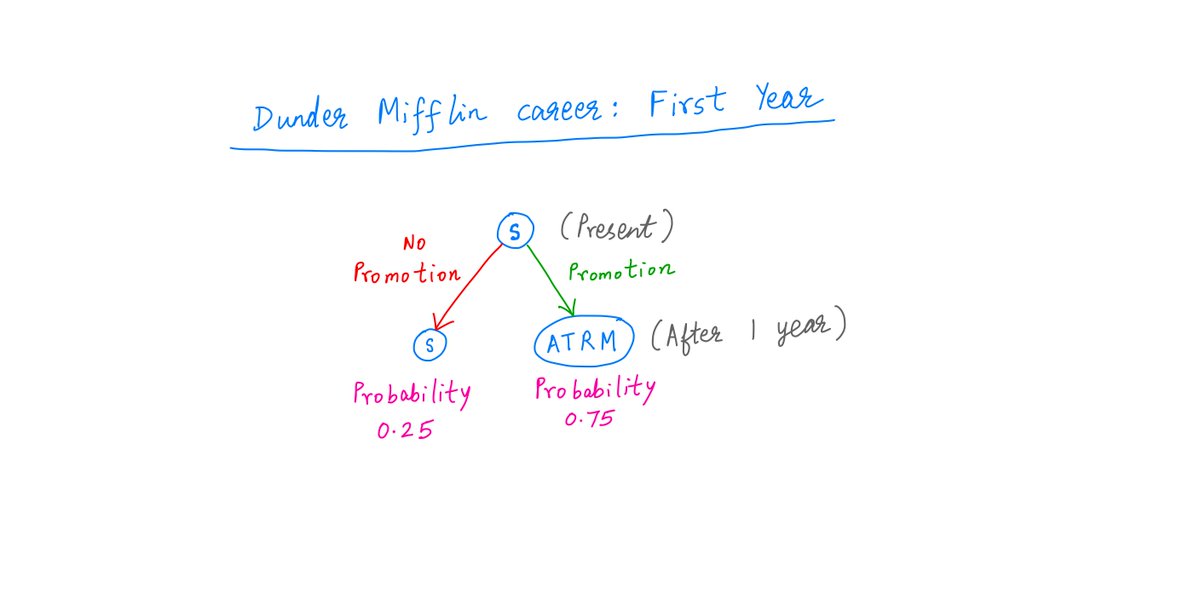
At the end of your first year on the job, you come up for a promotion.
If you get it, you become Assistant To the Regional Manager (ATRM). If not, you remain Salesman (S). The odds are 75/25.
Let's tackle this one year at a time.
At the end of your first year on the job, you come up for a promotion.
If you get it, you become Assistant To the Regional Manager (ATRM). If not, you remain Salesman (S). The odds are 75/25.
12/
So, at the end of Year 1, there are 2 possible states you could be in: S (Salesman) and ATRM (Assistant To the Regional Manager).
S has a 25% probability and ATRM has a 75% probability.
So, at the end of Year 1, there are 2 possible states you could be in: S (Salesman) and ATRM (Assistant To the Regional Manager).
S has a 25% probability and ATRM has a 75% probability.
13/
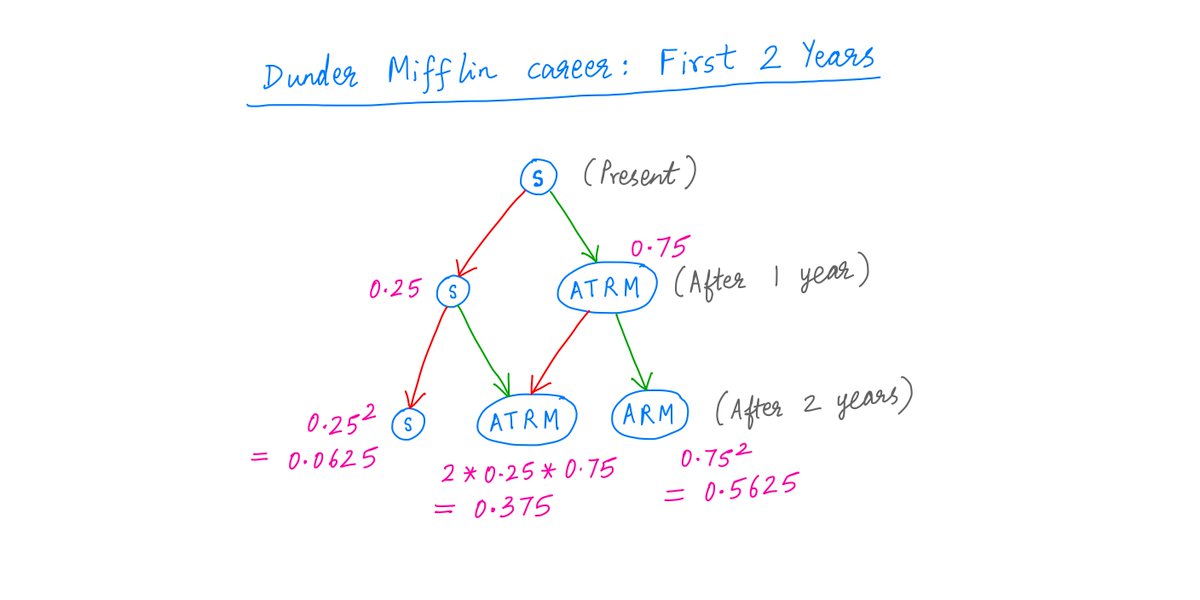
Similarly, at the end of Year 2, you again come up for a promotion.
Depending on whether you get it, there are now 3 possible states you could be in: S, ATRM, and ARM.
Again, each state has a probability (in pink below):
Similarly, at the end of Year 2, you again come up for a promotion.
Depending on whether you get it, there are now 3 possible states you could be in: S, ATRM, and ARM.
Again, each state has a probability (in pink below):
14/
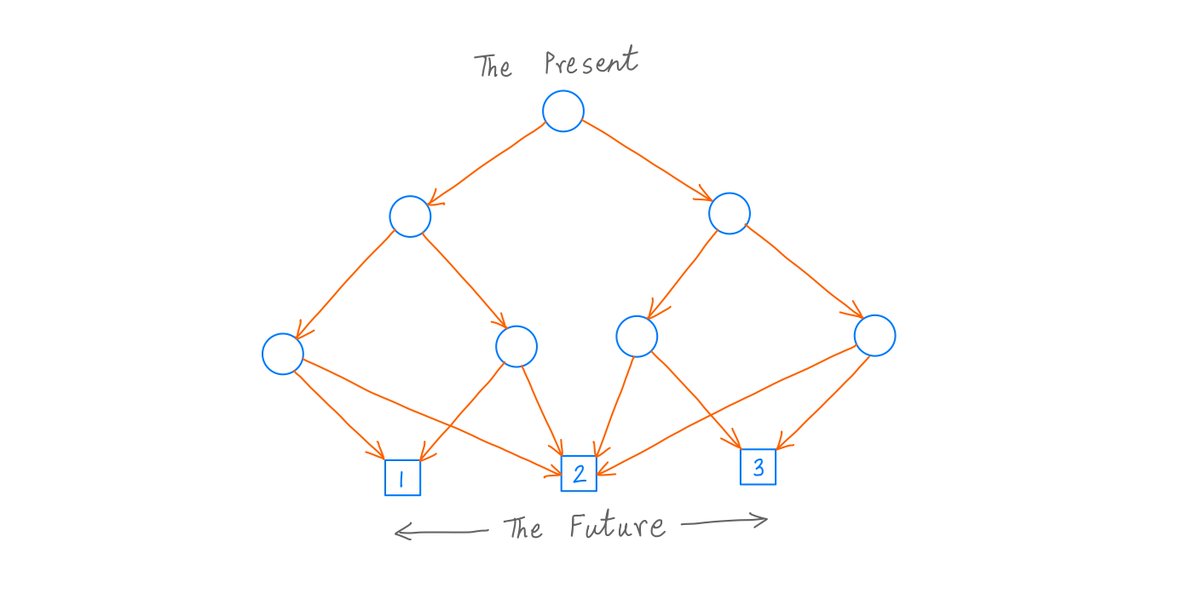
Continuing this way, we can draw up all the career trajectories you can possibly follow during your first 6 years at Dunder Mifflin.
In some of these trajectories, you achieve your goal of becoming CEO. In others, you don't.
Continuing this way, we can draw up all the career trajectories you can possibly follow during your first 6 years at Dunder Mifflin.
In some of these trajectories, you achieve your goal of becoming CEO. In others, you don't.

15/
And by simply propagating the 75/25 probabilities all the way down, we find that your probability of becoming CEO within 6 years is ~53.39%:
And by simply propagating the 75/25 probabilities all the way down, we find that your probability of becoming CEO within 6 years is ~53.39%:
16/
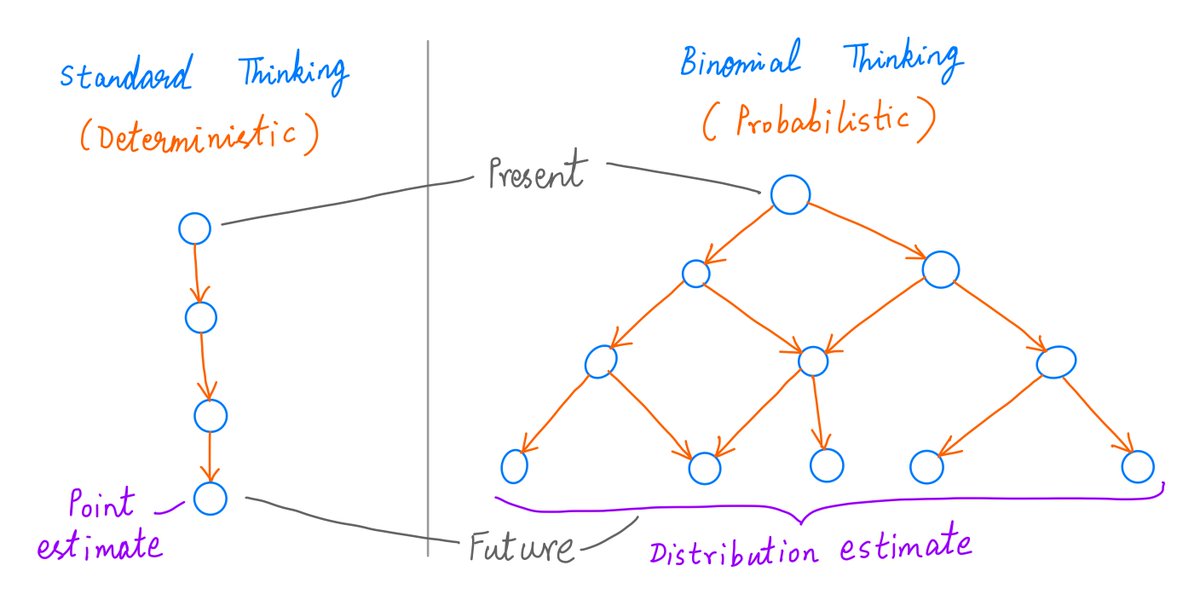
This example illustrates some key features of binomial thinking.
Feature 1. Break time into small chunks.
For example, in this case, we broke your first 6 years at Dunder Mifflin into 6 1-year chunks.
This example illustrates some key features of binomial thinking.
Feature 1. Break time into small chunks.
For example, in this case, we broke your first 6 years at Dunder Mifflin into 6 1-year chunks.
17/
Feature 2. At the end of each time chunk, figure out all possible states we can be in.
For example, at the end of Year 2 at Dunder Mifflin, your possible states are: S, ATRM, and ARM.
Feature 2. At the end of each time chunk, figure out all possible states we can be in.
For example, at the end of Year 2 at Dunder Mifflin, your possible states are: S, ATRM, and ARM.
18/
Feature 3. At each possible state, consider 2 possible scenarios and where each one leads.
The scenarios could be getting a promotion or not. The S&P 500 going up or down. An election won by a Democrat or a Republican. A guilty or not guilty court verdict. Etc.
Feature 3. At each possible state, consider 2 possible scenarios and where each one leads.
The scenarios could be getting a promotion or not. The S&P 500 going up or down. An election won by a Democrat or a Republican. A guilty or not guilty court verdict. Etc.
19/
Feature 4. Account for probabilities. Propagate them top-down through the binomial diagram to work out the chances of getting various desirable and undesirable outcomes.
Feature 4. Account for probabilities. Propagate them top-down through the binomial diagram to work out the chances of getting various desirable and undesirable outcomes.
20/
That's pretty much all there is to binomial thinking.
As you've seen, it's a simple way to incorporate chance events and probabilistic outcomes into our analyses.
It's particularly useful when simple point estimates and range estimates prove to be inadequate.
That's pretty much all there is to binomial thinking.
As you've seen, it's a simple way to incorporate chance events and probabilistic outcomes into our analyses.
It's particularly useful when simple point estimates and range estimates prove to be inadequate.
21/
But there are also drawbacks to binomial thinking.
For example, it advocates a binary worldview. At each state, we only account for 2 possible ways the future can unfold (eg, "promotion" vs "no promotion").
But there are also drawbacks to binomial thinking.
For example, it advocates a binary worldview. At each state, we only account for 2 possible ways the future can unfold (eg, "promotion" vs "no promotion").
22/
But often, there are more than 2 ways.
For example, the S&P 500 may go down 30%, up 5%, up 25%, etc. The possibilities are endless, but binomial logic reduces them to just 2.
Also, in many situations, the binomial diagram becomes pretty big -- and hard to analyze.
But often, there are more than 2 ways.
For example, the S&P 500 may go down 30%, up 5%, up 25%, etc. The possibilities are endless, but binomial logic reduces them to just 2.
Also, in many situations, the binomial diagram becomes pretty big -- and hard to analyze.
23/
But even with these drawbacks, binomial thinking is a definite step up over standard deterministic thinking.
In the land of the blind, the one-eyed man is king.
In the land of point estimators, the binomial thinker is king.
But even with these drawbacks, binomial thinking is a definite step up over standard deterministic thinking.
In the land of the blind, the one-eyed man is king.
In the land of point estimators, the binomial thinker is king.
24/
Many financial calculations rely on binomial thinking. Examples include the binomial options pricing model and its cousin Black-Scholes.
Also, this paper by @mjmauboussin uses binomial thinking to value the "optionality" of businesses: capatcolumbia.com/Articles/FoFin…
Many financial calculations rely on binomial thinking. Examples include the binomial options pricing model and its cousin Black-Scholes.
Also, this paper by @mjmauboussin uses binomial thinking to value the "optionality" of businesses: capatcolumbia.com/Articles/FoFin…
25/
At every tweet of this thread, you had a binary choice: you could continue reading, or you could skip the rest of the thread.
You're that special person who elected to continue 25 times in a row. A binomial wonder.
Thank you so much!
Have a great weekend.
/End
At every tweet of this thread, you had a binary choice: you could continue reading, or you could skip the rest of the thread.
You're that special person who elected to continue 25 times in a row. A binomial wonder.
Thank you so much!
Have a great weekend.
/End
• • •
Missing some Tweet in this thread? You can try to
force a refresh