Tantrasaṅgrāha was composed in 1500 CE in Sanskrit at about the time Jyeṣṭhadeva- who authored Yuktibhāṣā was Born
@JoeAgneya


Sinθ′−Sinθ = Cosθ(θ′−θ) where (θ′−θ) tends to 0
Thus,we can indeed say the Principles of Integral Calculus were first stated in Highly-Sanskritised Malayalam in Yuktibhāṣā
Given that the sources of the excerpts have been inquired of-the details regarding the same are in the tweet below
Do access the books if possible, online : )
In Sanskrit,Jyā and Kotijyā of a Dhanu(Arc) correspond to Sine and Cosine-here they are Functions of an Arc than an Angle

Did the Hindu Astronomers indeed know of Differential Calculus ?
They formulated Differential Forms of a few functions like the Sine Function,but did they Demonstrate an Idea of Limits ?
Did they formulate the Difference Quotient of Calculus ?
Spaṣṭādhikāra deals with the Rectification by Bhāskara- of Calculations on Planetary Motion made by Astronomers who preceded Bhāskara
Calculation of the Time of Eclipses,Alignment of Planets and Occultation of Stars was of Vital Importance to deliberate on Tithis and Kālas within a Tithi
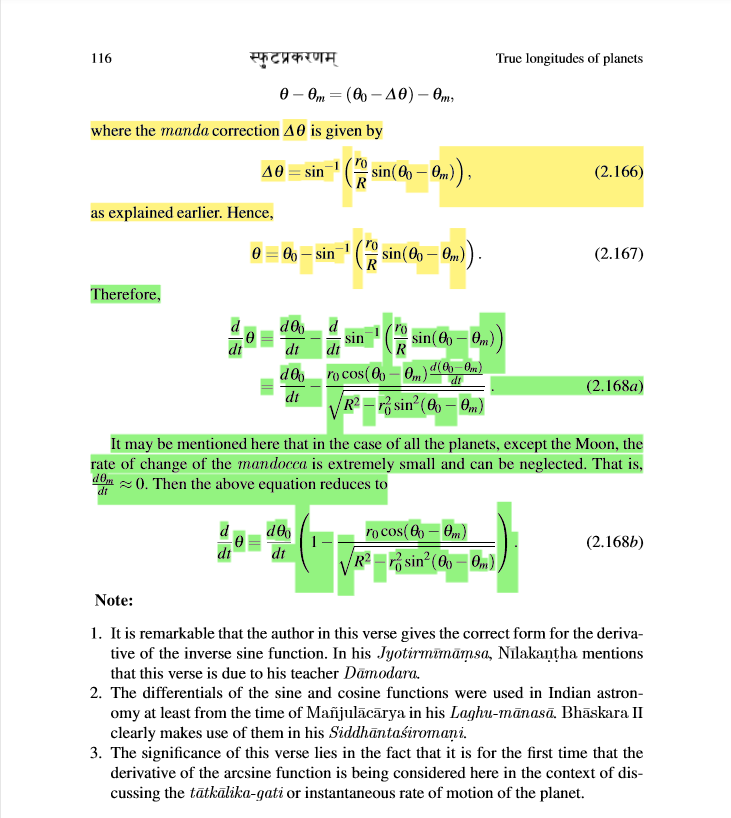
This motivates the Determination of the True Instantaneous Motion of a Planet/Star at any Particular Moment
Hindu Astronomers referred to this Instantaneous Motion at a Given Moment as Tātkālikagati

How does something move or possess velocity at a particular moment in time ?
What do motion or velocity even mean when time is frozen-when time is a constant and thus change in time or differential of time or dt=0 ?
Rather than describing motion when time is frozen/at an instant- can we define motion and velocity between intervals of time when such intervals are infinitesimal in nature ?
Bhāskara II thus formulates the dichotomous terms of Sthūlagati and Tātkālikagati meaning Gross and Instantaneous Motions respectively
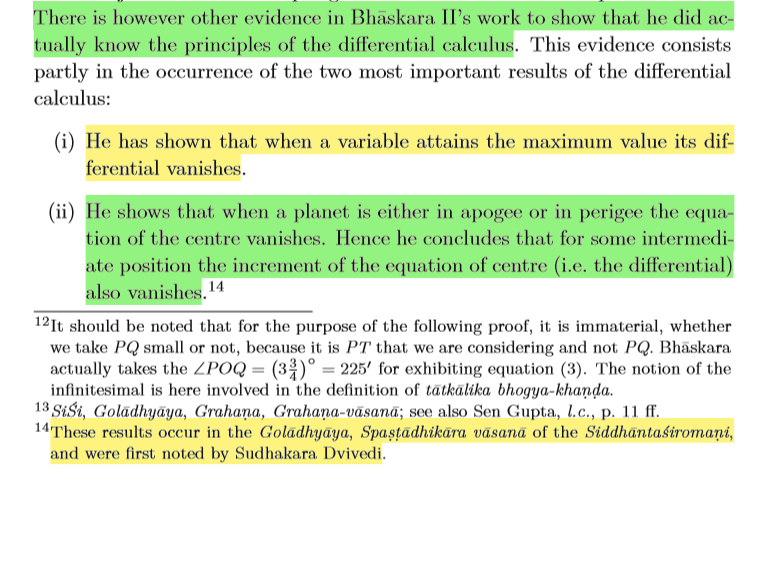
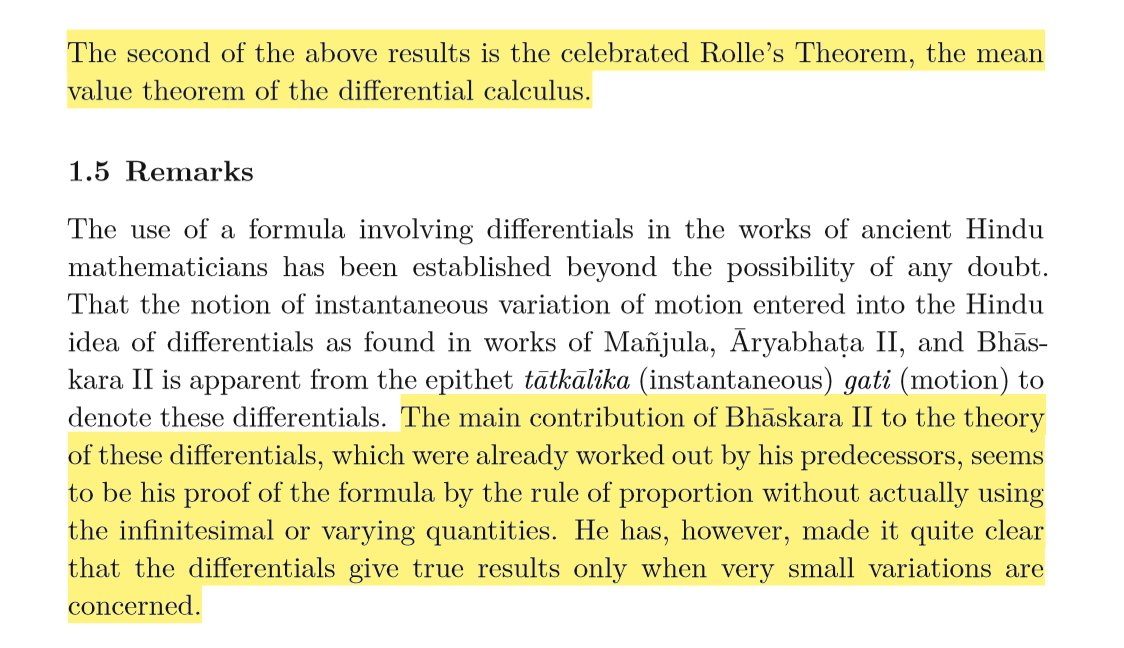
Bhāskara II not only mentions the Differential of Sine which he labels as Tātkālika Bhōgya-Khaṇḍa but also its Derivation and Proof
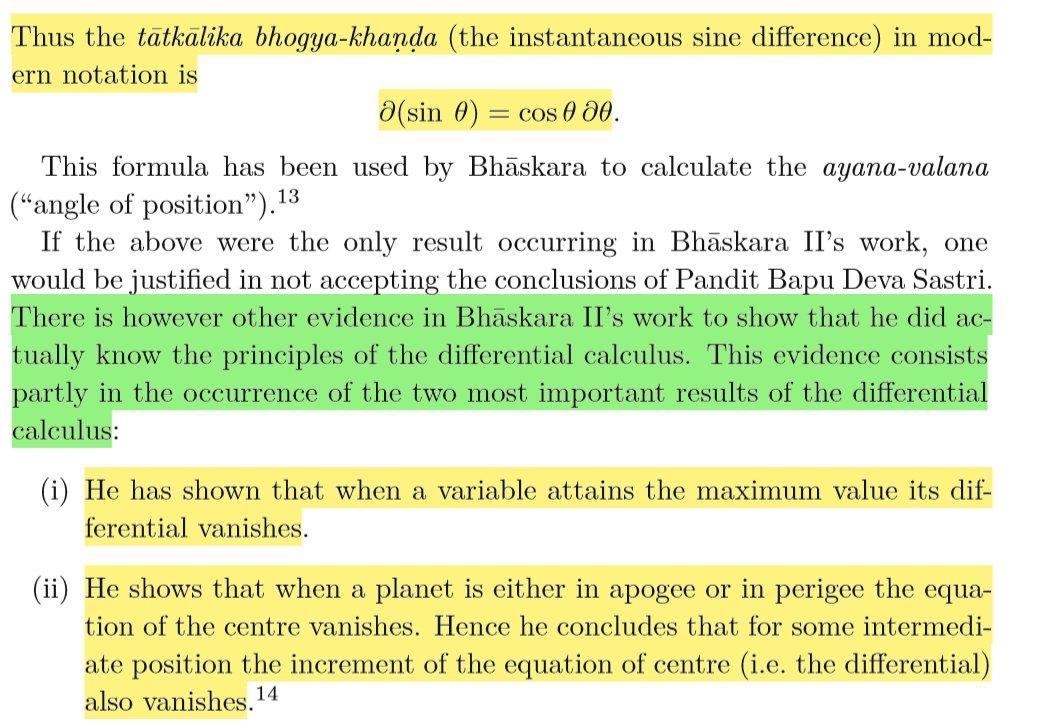

Bhāskara also demonstrates that the Maxima and Minima of a Function occur when its Differential/First Derivative is Zero